Grid reference finder
Improve your map reading skills by learning how to read a 4-figure, 6-figure or 8-figure national grid reference. This grid reference finder is suitable for beginners and includes a short ‘how to’ video.
Find grid references online
The quickest way to find a grid reference of a location is to go to the OS Maps desktop version and right click on the location – it’s that simple! You should see two letters followed by two sets of five numbers like this SZ 65750 98047. If you’re using the OS Maps app then you’ll need to press and hold to find the grid reference for that location. It will follow the same format as the above.
Another great grid reference finder is the OS Locate app which you can use to pinpoint your exact location.
Of course, we all know there’s nothing more reliable than a paper map and compass when venturing out into the hills. So, it’s important to know how to find your location (grid reference) on a paper map to let others know (like Mountain Rescue) know where you are. To do this, you need to understand the National Grid.
Find grid references with a paper map
Understanding the National Grid
Eastings and Northings
You might have noticed by now that OS Maps are covered in a series of blue grid lines. These grid lines help you to pinpoint an exact location anywhere on the map. The vertical lines are called ‘eastings’, as they increase in value as you travel east on the map. The horizontal lines are called ‘northings’ as they increase in value as you travel north on the map.
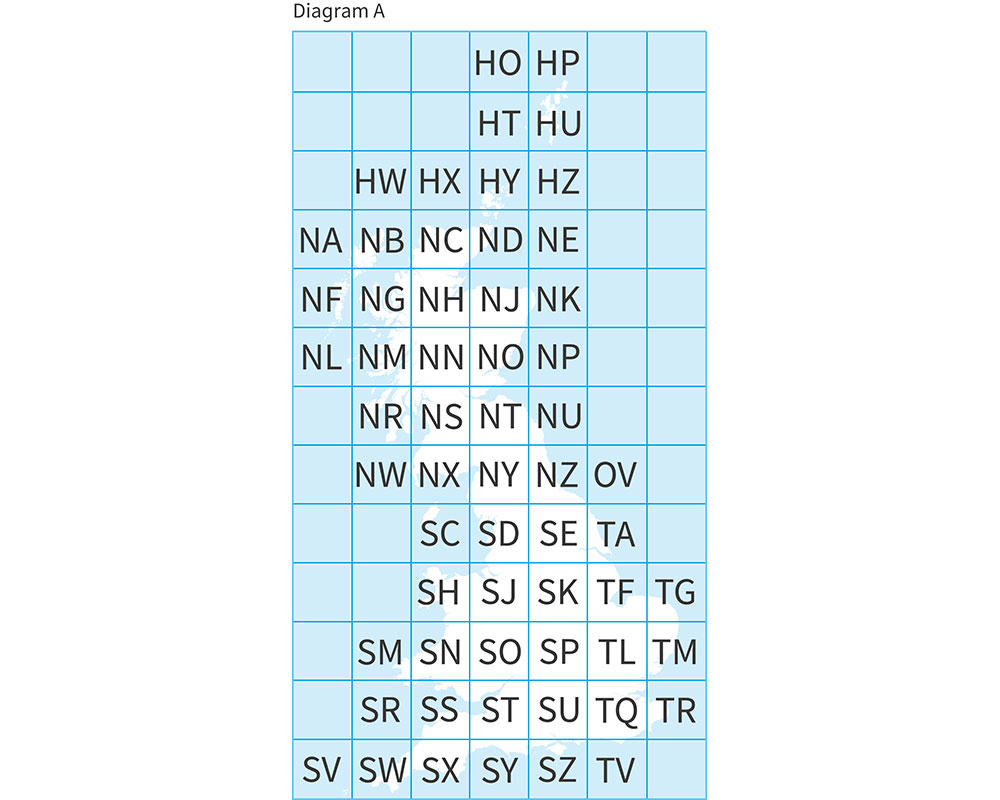
These are linked to the National Grid which provides a unique reference system, and can be applied to all OS Maps of Great Britain, at all scales. Great Britain is covered by grid squares measuring 100 kilometres across and each grid square is identified by two letters, as shown in diagram A.
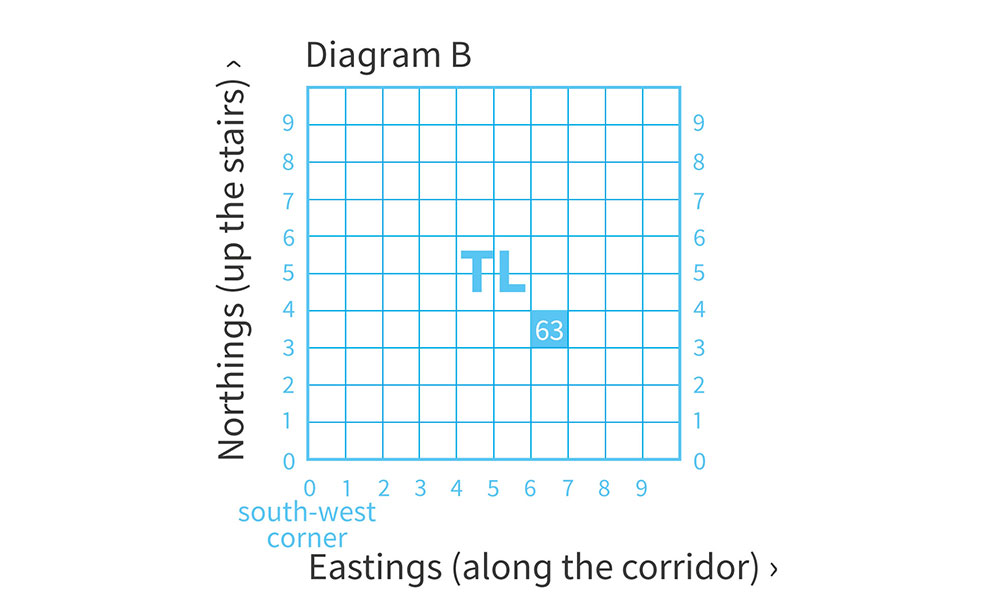
On OS Maps, these squares are further divided into smaller squares by grid lines representing 10 kilometre spacing, each numbered from 0 to 9 from the south west corner, in an easterly (left to right) and northerly (upwards) direction. You can see this in diagram B.
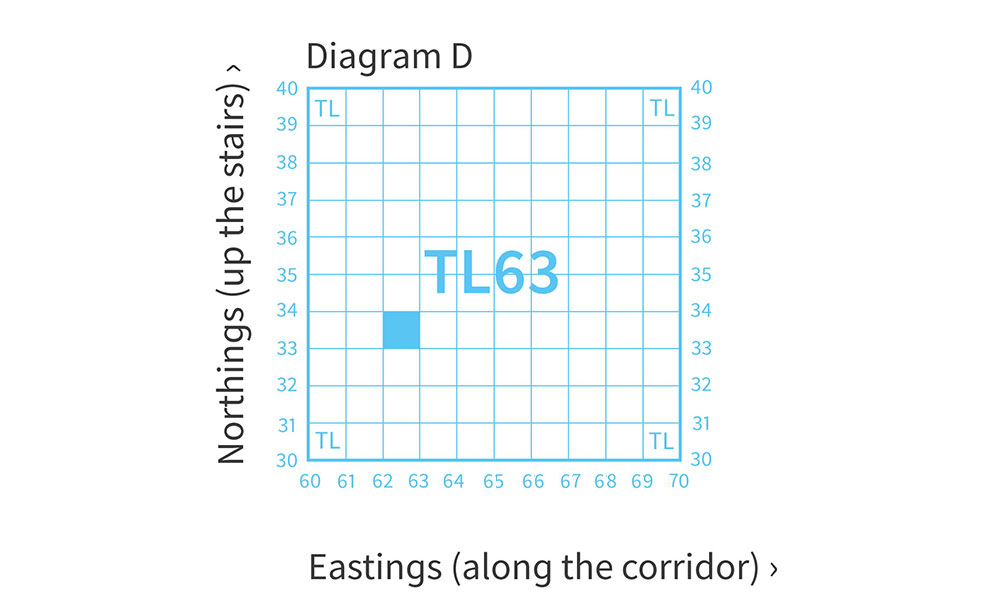
Using this eastings and northings system you can identify a 10 kilometre grid square. For example, the above image shows TL63. After the letters you can quote the eastings (6) first, then the northings (3).

Top Tip
If you have trouble remembering the order, say… along the corridor, THEN up the stairs.
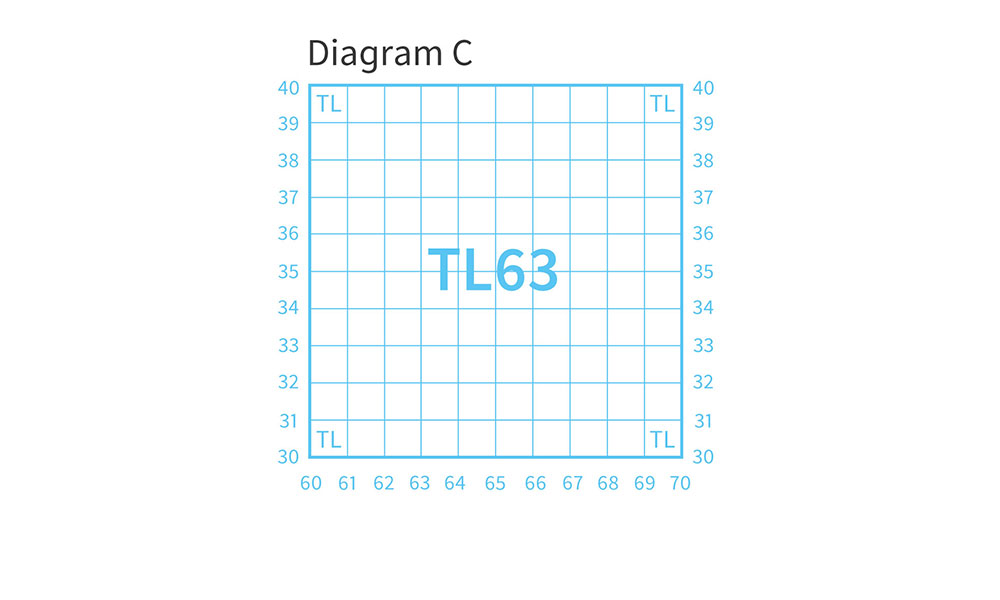
On an OS Landranger map you can find the two main grid letters (in this case TL) on the legend or the corner squares of the map. The grid is further divided into 1 kilometre intervals, as shown in diagram C.
How do grid references help me find places?
If you haven’t got OS Maps or OS Locate or you’re using a paper map, it is easy to find a particular place using a grid reference. To start, a four-figure grid reference is a handy way of identifying any square on a map. Grid references are easy if you can remember that you always have to go along the corridor before you go up the stairs. To find the number of a square first use the eastings to go along the corridor until you come to the bottom left-hand corner of the square you want.
Write this two-figure number down. Then use the northing to go up the stairs until you find the same corner. Put this two‑figure number after your first one and you now have the four-figure grid reference, which looks like the example in diagram D: 6233.
4-figure grid references
What this short ‘how to’ video with Steve Backshall
Six-figure grid references
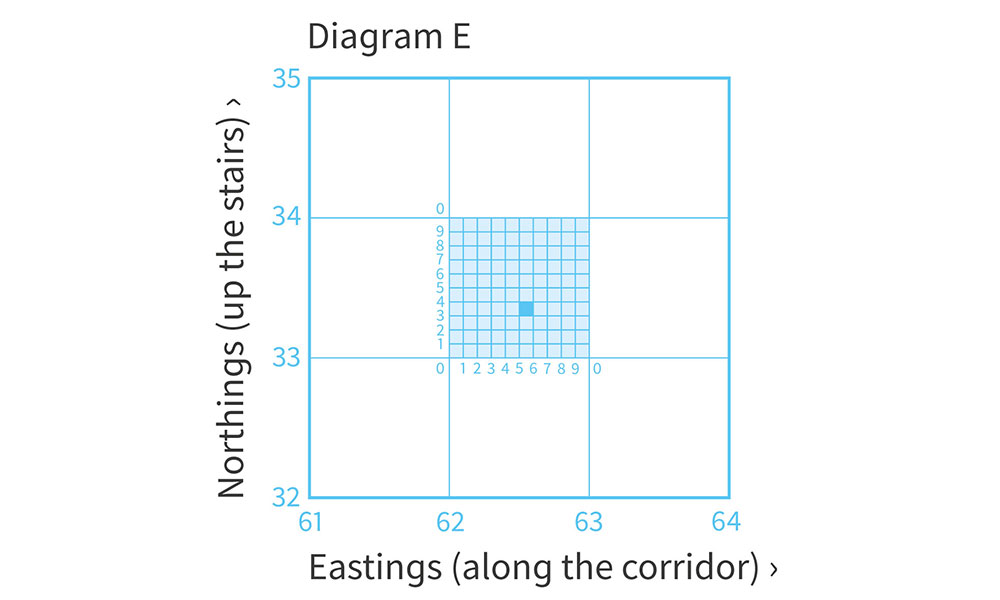
If you want to pinpoint an more exact place on a map, such as your own house, you will need to use a six-figure grid reference. First find the four-figure grid reference for the square and write it down with a space after each set of numbers, like this: 62_ 33_
Now imagine this square is divided up into 100 tiny squares with 10 squares along each side. Still remembering to go along the corridor and up the stairs, work out the extra numbers you need and put them into your four-figure grid reference like this in diagram E: 625 333.

Top tip
When giving directions you can provide even more accuracy to your grid reference by stating a nearby landmark or feature. For example, on the Bembridge OS Explorer map I am at grid reference SZ 644 874, at the crossroads.
What this short ‘how to’ video with Steve Backshall
Put your new skills into practice and get out your OS Map to have a go at finding a few grid references. Check out our Pathfinder guide titled Navigation Skills for Walkers including map reading, compass and GPS.